 |
Vol X No. 1 Spring |
||||||||||||||||||
 Photo credit - Rebecca Gammill
As I begin preparations for the next school year, I am reflecting on the changes that have taken place in mathematics classrooms in the seven years that I have been working with districts in the North Georgia RESA area and with educators across the State. During this time we have transitioned from the Georgia Performance Standards (GPS) to Common Core Georgia Performance Standards (CCGPS) and now to the Georgia Standards of Excellence (GSE). This has brought about changes to students ' behavior in the classroom, teachers' instructional methods, and my own mathematical thinking. I often think about my middle school students and hope that I did not damage them or cause unnecessary anxiety for them. I know I am a much better teacher today because of my personal growth and many conversations with colleagues.I now realize that I am not required to know all the answers. I blame this expectation on my nephew, Hunter. When he was in elementary and middle school, he would say, "My Aunt Bonnie is a teacher and she knows everything!" Talk about pressure! I understand that it is okay for teachers to say, "I don't know", "I am not sure", and "Let me think about this more." Teachers' mindsets are moving from the idea that we must give students all the information they need up front and have them work independently. Students are now given problems to solve, expected to ask for the information they need, and allowed to work with other students to develop a solution instead of depending on the teacher for the one correct answer and strategy. We understand that constant assessment of students to adjust instruction is more efficient than waiting for the "end-of- unit" test to uncover what needs to be retaught. Students are more engaged in the mathematics because problem solving is active, not passive. Allowing students to work together appeals to the social nature in all of us. Having students explain their thinking and justify their answers and strategies gives ownership of the learning back to the students. I would have been a much better math student and problem solver if I had been taught this way. Teacher collaboration certainly contributes to this professional growth. I have learned how to help students make connections between mathematical ideas through rich mathematical discourse. For example, one teachers may create open tasks that require students to find patterns and describe how they see these patterns (using dot cards during Number Talks, for example) instead of simply determining the 100 th pattern or finding the equation. This type of task allows for differentiation that provides access to all students --"low floor and high ceiling". As adults, we get excited when we make these connections. How may we create atmospheres for learning that allow students to experience this excitement for themselves? For more ideas, professional connections, and mathematical discourse of how to engage your students in mathematics, consider attending the Summer Academies offered by GCTM. Information about the Summer Academies is provided below.
All over the state of Georgia this summer, teachers will be gathering together to improve their mathematical pedagogy! Once again GCTM will be hosting the Summer Mathematics Academies in three different locations. You have the opportunity to join a professional learning session for two days with peers that teach in the same grade-band as you. Along with a focus on creating quality mathematical discourse in your classroom, you will have the opportunity to collaborate with others. Teachers helping teachers! As one participant from last summer said that the best thing about the Summer Academies was "getting to talk to other teachers who face the same struggles that I do in the classroom."
Facilitators working in the area of mathematics every day will host the following sessions:
K-1st Grade These sessions will focus on quality tasks that model effective math teaching practices. The facilitator will demonstrate and teach the participants how to set clear goals, anticipate how the students will solve a problem and how to use this anticipation to deliberately create mathematical discourse in the classroom. Nothing screams high-quality math lesson better than when the students have the opportunity to share and discuss their learning by explaining how they solved the problem. During the sessions this summer, teachers will also learn how to sequence when students share to get the most learning and growth from the class of students. At times, students will better understand the lesson if a misconception is shared first. However, other lessons will benefit the most from students sharing less sophisticated strategies first. During the 2018 Summer Mathematics Academies, participants will learn the importance of sequencing the share time portion of the lesson. Using the strategies learned from the sessions this summer, teachers will learn to have more control over the learning of their students while developing quality discussions between and amongst students.
A new introduction to last year's Academy was the Lunch-N-Learn! Summer Academy participants had the chance to sign up for an extra class taught during lunch. Each participant was given a free lunch while they learned about 3 Act Tasks. This activity was so successful that we are working to increase the opportunity for both days of the Academies! Participants will have the opportunity to gain extra knowledge during lunch both days!
Free, free, free is every teacher's favorite word, especially if it pertains to classroom materials! Many participants were lucky enough to win FREE classroom materials. Everything from teacher books to free manipulatives were given away at each Academy! The winners were so excited to have something extra to take back with them to the classroom.
If you are still uncertain about spending two days this summer increasing your mathematical pedagogy, then see what others had to say about the 2017 Summer Mathematics Academies!
Join us for the 2018 Summer Mathematics Academies where we will become greater than the sum of our separate effects!
K-1st, 2nd-3rd, 4th-5th, 6th-8th, Algebra, Geometry, and High School STEM using the TI-Inspire
E-mail questions to academies@gctm.org
GCTM Hosts Math Day at the State Capitol On February 13th, 2018 a group from the Georgia Council of Teachers of Mathematics travelled from across the state to Atlanta for Math Day at the Capitol. The day began in the Senate Chamber where Senate Finance Committee Chairman Chuck Hufstetler (R-Rome) recognized GCTM with a resolution and invited Bonnie Angel to address the members of the Senate. This provided an opportunity for Bonnie to thank the members of the Senate for their support of mathematics teachers across the state and remind them of the critical work that GCTM does to support quality mathematics instruction in Georgia. The group then hosted a lunch in the capitol where numerous key influencers (listed below) came by to learn more about GCTM's role in Georgia and discuss key education issues that are facing the state. This provided a critical opportunity to update these influential leaders on the work of GCTM's members, thank them for their leadership, and discuss relevant legislation that is pending in the House/Senate. The Georgia Council of Teachers of Mathematics would like to extend its sincere thanks to the following leaders who came out for the math day lunch and took time out of their day to speak to our group. We are grateful for your partnership and look forward to continuing to work with you to promote high quality mathematics education in Georgia! Senator Brian Strickland (R-McDonough)
Legislators Attend GCTM Events Throughout Georgia Representative Wes Cantrell (R-Woodstock) visited Clark Creek Elementary School on October 26th during which time school administrators and GCTM leadership spent the morning briefing him on the work that goes into preparing teachers for the classroom, supporting their ever-changing needs, and ensuring all students are given the tools they need to be successful. Rep. Cantrell is a Subcommittee Chairman on the House Education Committee and plays a key role in shaping education policy in Georgia. The classroom visit provided him with an opportunity to spend quality time with mathematics teachers learning about the challenges they face and engaging in meaningful discussions about how local and state policies affect their work. GCTM is grateful to Representative Cantrell for the time that he spent with the group and for his willingness to learn about what our members do each and every day. Below are some pictures from the visit to Clark Creek Elementary School:
Save the Date! Check your calendar to make sure that you have set aside these special days this fall --Oct. 17, 18, and 19 -- to participate in what may be one of the most profound mathematical experiences of your school year! The 2018 Georgia Mathematics Conference will focus on how wrestling with mathematics is critical to building strong and healthy math minds through the theme "Embracing Productive Struggle." Immerse yourself in the culture of a learning community during your time at Rock Eagle as you participate in engaging sessions, conversations with experts and colleagues from around the state and nation, and other activities after a long day of strenuous (but gratifying!) learning. For those of you who are able to arrive at Rock Eagle on Wednesday, there will be a special event occurring that afternoon that ...let's just say fits in well with the conference theme and that we think you will enjoy!Keynote Speakers The keynote speakers for this year's conference will include Thomasenia Adams, Matt Larson, and James Tanton.
Dr. Adams engages in mathematics education through mentoring future mathematics educators, presenting at conferences, facilitating professional development across the US and Canada, and publishing articles and books. She is the co-author of the Solution Tree series Making Sense of Mathematics for Teaching, Grades K-2 and Grades 3-5 (2016) and Beyond the Common Core: A Handbook for Mathematics in a PLC at WorkTM, Grades K-5 (2012). Furthermore, Dr. Adams is a leader of Dixon Nolan Adams Mathematics professional development team, along with colleagues Juli Dixon and Ed Nolan. Having served as a mathematics coach for Grades K-12, Dr. Adams has a deep respect for what it means to teach mathematics for understanding.
Larson is a frequent speaker before mathematics education audiences, and he has authored or co-authored several books, including a series on professional learning communities and Common Core Mathematics. He is co-author of Balancing the Equation: A Guide to School Mathematics for Educators and Parents, and he was on the writing team of Principles to Actions: Ensuring Mathematical Success for All (NCTM, 2014). Larson has taught mathematics at the elementary through college level and has held an appointment as an honorary visiting associate professor at Teachers College, Columbia University. In 1994 he was recognized for his teaching accomplishments with a U.S. West Outstanding Teacher Award.
James has written a number of books, including THE ENCYCLOPEDIA OF MATHEMATICS (Facts on File, 2005) and two wordless puzzle books, WITHOUT WORDS and MORE WITHOUT WORDS (Tarquin, 2015) -- which have each been translated in Serbian -- and developed two DVD Great Courses (The Teaching Company, 2013 and 2016). He advises on curriculum, consults with teachers, and gives demonstration classes and professional development across the globe. James also leads the MAA's Curriculum Inspirations project www.maa.org/ci and is a founder of The Global Math Project www.theglobalmathproject.org, an initiative to transform the entire world's perception of what mathematics can and should be. During the inaugural Global Math Week in October 2017, over 1.7 million students across the globe took part in a common joyous piece of mathematics and saw how classroom mathematics can serve as a portal for human joy, wonder, and delight. How will you be a part of this conference?
As a member of GCTM, you have a reserved seat at the table of making a difference in mathematics education. Will you take your place at the table at Rock Eagle this year? Whether as a presenter, a volunteer, a participant, or in some other capacity, we welcome you to come together for a time to do some meaningful mathematics. Go to GCTM's website, and select the GMC at Rock Eagle tab for the latest information as it becomes available! If you are interested in presenting a session at the conference this year, please think about how you, your students, and/or your colleagues have experienced productive struggle in mathematics and what you would like to share with others about your experience. Some of the best ideas and strategies come from you and your own neighbors right here in Georgia! Access this link to complete a speaker proposal form.
Unpacking CCSSM: Developing understanding of fractions as numbers
Figure 1. "Does the shaded region represent 5 /6 or 5/3? We cannot say unless the whole is specified." (Beckmann, 2014)Consider the visual in figure 1: Does the shaded region represent 5/6 or 5/3? In fact, the answer could be both of these fractions. The answer to this question depends on the individual student's choice of the whole.
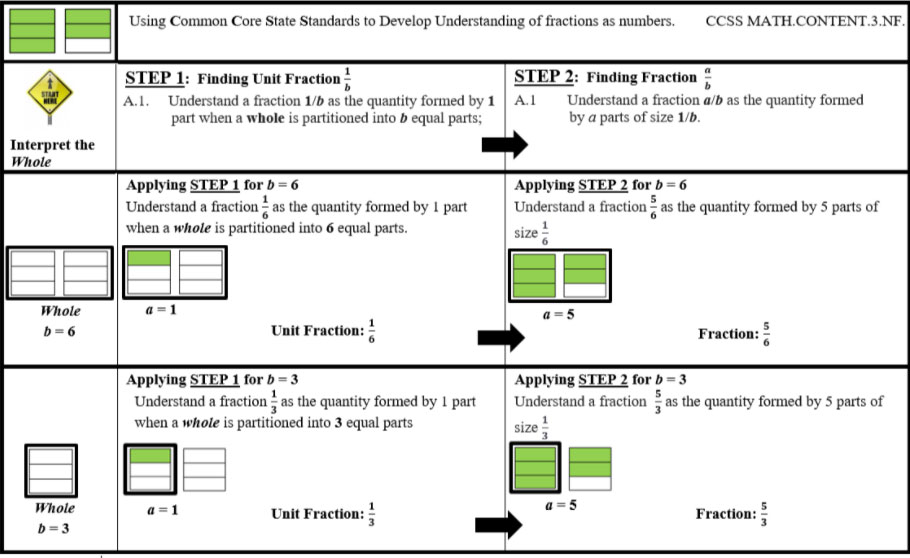
Figure 2. CCSSM: Developing understanding of fractions as numbers. CCSSM expects mathematically fluent third-grade students to develop an understanding of fractions in terms of unit fractions (fig. 2) and to begin to see fractions as numbers (CCSSI 2010). When given a fraction, third-grade students should be able to use CCSS.MATH.CONTENT.3.NF.A.1 to represent the fraction with a picture or scenario. When given a picture or scenario, does this mean that students can use this standard to arrive at a unique fraction? The answer to this question is yes, but with one important condition. The student must first choose the whole. To address this, let's revisit the shaded region shown in figure 1. Based on the student's interpretation of the whole, CCSS.MATH.CONTENT.3.NF.A.1 (see fig. 2) can be correctly applied to obtain two different fractions. As table 1 reveals, the student first interprets the whole as the area of either one large rectangle or the area of both large rectangles and then uses the standard to interpret the unit fraction 1/b as the area of one part (shaded in green) when the area of the whole is divided into b parts of equal area (Step 1). Notice that both 1/6 and 1/3 could be considered correct fractions according to the student's interpretation of the whole. After the unit fraction is determined, the student then uses this to either get 5/6 or 5/3 for the fraction a/b represented by the area of five of the equal parts shaded in green (Step 2).
As shown above, unless the whole is made explicit, the fractions matching the figures can be interpreted in different ways. Therefore, we suggest that students should intentionally state the whole as the first step prior to naming a fraction corresponding to a given figure. Once the whole is specified, students can confidently follow the standard (see fig. 2) in order to arrive at a unique fraction. Fraction Tasks with Purposeful Ambiguity Seemingly counterintuitive, in order to help our PSTs arrive at an understanding of the importance of the whole, we decided to introduce the ambiguity found in step 1 in Table 1. Not only does "ambiguity allow students to discuss deep, rich mathematical ideas" (Danielson, 2016), but it also promotes meaningful mathematical discourse (NCTM, 2014). Thus, we created Task 1 (see fig. 3).
Figure 3. Task 1 After a few minutes attempting Task 1 individually, students put 1/6 or 1/3 as their answers, and many did not include units in their responses. Some student work was selected and displayed in Figure 4.
Figure 4. Student Work on Task 1. Once the PSTs completed the task, they were encouraged to share their answers with the whole class. The conversation went as follows.
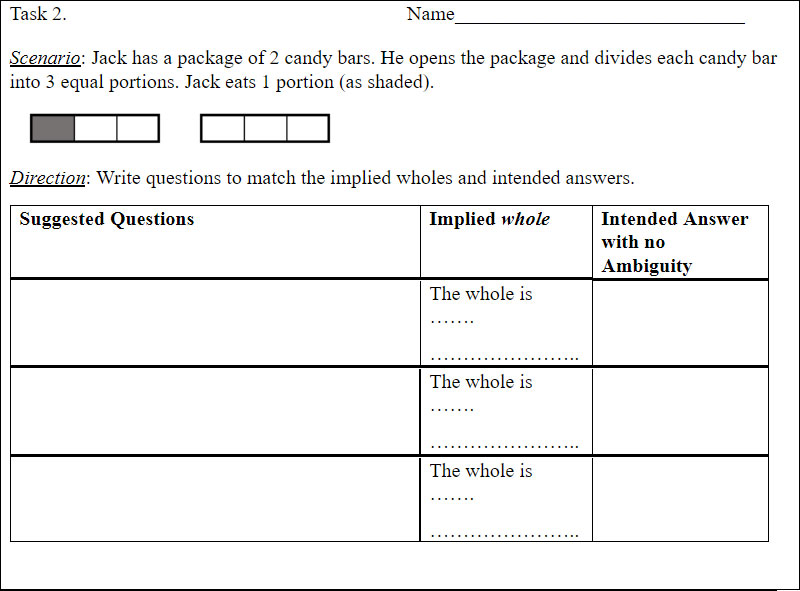
An "aha moment" occurs when the students realize that once the whole has been defined, the ambiguity disappears. The question ("How much does Jack eat") in Task 1 is purposely vague and consequently elicits ambiguous answers. After all, what exactly does "how much" mean? What if the question in Task 1 is asked more explicitly? Would this be less confusing for students? In order to address this, students were put in groups to work on Task 2 (see Fig. 5).
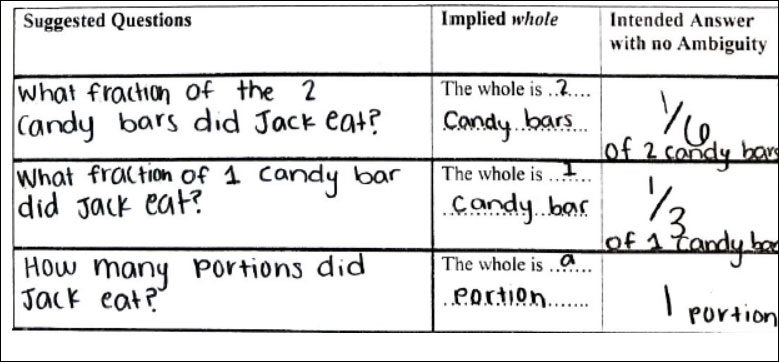
Figure 5. Task 2. The purpose of Task 2 is for students to recognize the importance of the whole when working with fractions. In this task, students in each group were asked to write a question to match each of the intended answers they proposed for Task 1 and to identify the implied whole in each case. Student responses on Task 3 were shared with the class, and a student's work was selected to display in Figure 6. After working in groups and generating these suggested questions, students gained a clearer interpretation of the whole so that they will be more apt to first determine whether the whole in reference to the question is one object (a candy bar) or a collection of objects (two candy bars) prior to naming a fraction in response to a given problem.
Figure 6. Student Response on Task 2. Student Reflections on Tasks 1 and 2 After completing Tasks 1 and 2 in class, the PSTs reflected on what they learned from working on the tasks by filling out a Google Form on their own electronic devices. Overall, students expressed that they learned the importance of identifying the whole before naming a fraction as well as why they should include both numbers and units in their answers. Some said that at first they assumed either 1/3 or 1/6 to be the answer to Task 1 (Fig. 3), but then they realized that the answer could be different, depending on the chosen whole. Many students stated that the tasks helped them foresee issues that may arise in their future classroom when they become teachers. Several students mentioned how helpful it was to work in groups and how much they learned from other students. A sample of student reflections on two tasks is shown in Figure 7.
Figure 7. Student Reflections on Learning from Tasks 1 and 2 Closing Thoughts Reflections from students showed that the Tasks 1 and 2 (Figures 3 & 4) not only helped them see the importance of identifying a whole when learning fractions and why they should include units in their answers but also allowed them to work backwards to design questions to match their "intended answer with no ambiguity." Moreover, they indicated that they learned from others through group discussion. In conclusion, we would like to make the following suggestions to teachers. First, have students identify the whole prior to determining a fraction from a picture or word problem (see CCSS.MATH.CONTENT.3.NF.A.1 in fig. 2). In addition, students should include units with their answers. Finally, when working with fractions, pose problems that introduce ambiguity so that students will have conversations concerning the importance of the whole. Otherwise, make the whole in reference to the question explicit. For instance, ask "What part of a candy bar did Jack eat?" or "What fraction of the package of candy bars did Jack eat?" We encourage mathematics educators to try these tasks or create their own so that students will recognize the important role that the whole plays in understanding fractions. REFERENCES Beckmann, S. (2014). Mathematics for Elementary Teachers with Activities. 4th ed. New York: Pearson. Common Core State Standards Initiative (CCSSI). (2010). Common Core State Standards for Mathematics (CCSSM). Washington, DC: National Governors Association Center for Best Practices and the Council of Chief State School Officers. http://www.corestandards.org/wp-content/uploads/Math_Standards.pdf Danielson, C. (2016). The Power of Having More Than One Right Answer: Ambiguity in Math Class. Teaching Children Mathematics Blog. Retrieved from: http://www.nctm.org/Publications/Teaching-Children-Mathematics/Blog/The-power-of-having-more-than-one-right-answer_-Ambiguity-in-math-class/ Fennell, F (Skip). (2007). Fractions are Foundational. National Council of Teachers of Mathematics [NCTM] News Bulletin (December):1. Hecht, Steven A., Vagi, Kevin J., & Torgesen, Joseph K. (2007). Fraction skills and proportional reasoning. In Why is Math so Hard for Some Children? The Nature and Origins of Mathematical Learning Difficulties and Disabilities, edited by Daniel B. Berch & Michelle M. Mazzocco, pp. 121-132. Baltimore: Paul H. Brookes Publishing Co. Mazzocco, Michelle M., & Devlin, K.T. (2008). "Parts and holes: Gaps in rational number sense among children with vs. without mathematical learning disabilities." Developmental Science 11 (September): 681-91. National Mathematics Advisory Panel. (2008). Foundations for Success: The Final Report of the National Mathematics Advisory Panel. Washington, DC: U.S. Department of Education. Wu, Hung-Hsi. (2002). Chapter 2: Fractions (Draft). Retrieved from: https://math.berkeley.edu/~wu/EMI2a.pdf Wu, Hung Hsi. (2014). Teaching Fractions According to the Common Core Standards. University of California, Department of Mathematics. Retrieved on September, 2016 from: http://math.berkeley.edu/~wu/. Dr. Ha Nguyen is an Assistant Professor of Mathematics Education at Georgia Southern University. She has taught mathematics courses and mathematics content courses for pre-service teachers as well as providing professional development for K-12 in-service teachers in Georgia. She is interested in students' understanding and thinking of mathematics and how to make mathematics relevant to students. Dr. Joy Darley currently has the title of Associate Professor of Mathematics Emerita at Georgia Southern University and serves on the executive board for GCTM. Prior to her retirement in 2016, she taught mathematics content courses for pre-service teachers at Georgia Southern University. She is interested in both students' and pre-service teachers' conceptual understanding of mathematics, especially fractions and the arithmetic to algebra connection.
The GCTM Middle School Tournament will be held April 21, 2018 at Tattnall Square Academy in Macon ! Please consider bringing up to two teams of four middle school students have a fun day full of math! There are individual rounds as well as team events. The registration cost is only $10 for GCTM members! Please see the Competitions page of the GCTM website for the registration form and other information. The invitation-only GCTM-sponsored State Math Tournament will be April 28, 2018 at the Middle Georgia State University Conference Center in Macon. Invitations are based on tournament results in Georgia over the current academic year. Invitations will be issued out by April 2. For more on how invitations are determined, please see the Competitions page of the GCTM website. Are you interested in preparing students for these tournaments? All State Math Tournament problems from 1982 to 2015, and all Middle School Tournament problems from 2010 to 2015, are collected in books which are for sale on the GCTM website! (The first volume of State Math Tournaments is available as a free pdf!) See you in April!
Spring into GCTM Growth!
y
Renew your membership and remain a member! The small monetary cost will reward you exponentially and in turn have positive impact on your students and daily classroom activities. Membership remains at the 1200 level. But in searching some files this week I saw a record of 2900 members just 7 years ago. WOW! So why the decrease? What has changed? Let us know what you think. We still offer the following wonderful benefits.
We continue to work hard to reach out to you and your colleagues to make GCTM better and a vital asset to you. What can we do better? We are anxious to hear from you!
|
Table of Contents President's Message - by Bonnie Angel, GCTM President Synergy: A Combined Effect Greater than the Sum of their Separate Effects - by Kristi Caissie GCTM at the Capitol - by T.J. Kaplan, Advocacy GMC Update by Martha Eaves The WHOLE Truth About Fractions - by Ha Nguyen and Joy Darley GCTM Middle School Tournament - by Chuck Garner, VP for Competitions GCTM Membership Report - by Susan Craig, Membership Director NCTM Report - by Michelle Mikes
|
||||||||||||||||||
|
Georgia Council of Teachers of Mathematics | PO Box 683905, Marietta, GA 30068 |
|||||||||||||||||||













 Thomasenia Lott Adams, Ph.D., is associate dean for research and faculty development in the College of Education at the University of Florida. She holds the position of professor of mathematics education in the School of Teaching & Learning. Dr. Adams has been an active member of the National Council of Teachers of Mathematics for over 25 years and has served NCTM in a variety of roles (e.g., editor for the Mathematical Roots Department in
Mathematics Teaching in the Middle School, co-editor for the Investigations Department of
Teaching Children Mathematics). She is the Program Chair for the 2018 NCTM annual conference.
Thomasenia Lott Adams, Ph.D., is associate dean for research and faculty development in the College of Education at the University of Florida. She holds the position of professor of mathematics education in the School of Teaching & Learning. Dr. Adams has been an active member of the National Council of Teachers of Mathematics for over 25 years and has served NCTM in a variety of roles (e.g., editor for the Mathematical Roots Department in
Mathematics Teaching in the Middle School, co-editor for the Investigations Department of
Teaching Children Mathematics). She is the Program Chair for the 2018 NCTM annual conference. Matt Larson is president of the National Council of Teachers of Mathematics (NCTM), a 70,000-member international mathematics education organization. Previously, Larson was the K--12 curriculum specialist for mathematics in Lincoln (Nebraska) Public Schools for more than 20 years. His two-year term as NCTM president began in April at the conclusion of the 2016 NCTM Annual Meeting & Exposition in San Francisco.
Matt Larson is president of the National Council of Teachers of Mathematics (NCTM), a 70,000-member international mathematics education organization. Previously, Larson was the K--12 curriculum specialist for mathematics in Lincoln (Nebraska) Public Schools for more than 20 years. His two-year term as NCTM president began in April at the conclusion of the 2016 NCTM Annual Meeting & Exposition in San Francisco. James Tanton (Ph.D., Princeton 1994, mathematics) is an author, a consultant, and an ambassador for the Mathematical Association of America (MAA) in Washington D.C., currently serving as their Mathematician-at-Large. He has taught mathematics both at university and high-school institutions. James is absolutely committed to promoting effective and joyful mathematics thinking, learning, and doing at all levels of the education spectrum.
James Tanton (Ph.D., Princeton 1994, mathematics) is an author, a consultant, and an ambassador for the Mathematical Association of America (MAA) in Washington D.C., currently serving as their Mathematician-at-Large. He has taught mathematics both at university and high-school institutions. James is absolutely committed to promoting effective and joyful mathematics thinking, learning, and doing at all levels of the education spectrum.

 Proficiency with fractions is an important component for building the groundwork for learning more advanced mathematics (Wu, 2002; Fennell, 2007; NMAP, 2008; Wu, 2014). Yet, historically students of all ages seem to have difficulty with fractions (Hecht, Vagi, & Torgeson, 2007; Mazzocco & Devlin, 2008; NMAP, 2008). In 2010, the Common Core State Standards for Mathematics (CCSSM) (CCSSI 2010) were released to address the skills and knowledge all students need to know to be College and Career ready. Among these standards, some are designed specifically for developing an understanding of fractions as numbers. In order to reach this understanding, many mathematicians and mathematics educators agree that all fractions should be understood in reference to a unique whole (Wu, 2002; NMAP, 2008; Beckmann, 2014). In addition to unpacking CCSSM on
"developing understanding of fractions as numbers," the purpose of this paper is to share fraction tasks implemented in a class of K-8 preservice teachers (PSTs) with the intention of helping third-grade students to focus on the whole when learning fractions.
Proficiency with fractions is an important component for building the groundwork for learning more advanced mathematics (Wu, 2002; Fennell, 2007; NMAP, 2008; Wu, 2014). Yet, historically students of all ages seem to have difficulty with fractions (Hecht, Vagi, & Torgeson, 2007; Mazzocco & Devlin, 2008; NMAP, 2008). In 2010, the Common Core State Standards for Mathematics (CCSSM) (CCSSI 2010) were released to address the skills and knowledge all students need to know to be College and Career ready. Among these standards, some are designed specifically for developing an understanding of fractions as numbers. In order to reach this understanding, many mathematicians and mathematics educators agree that all fractions should be understood in reference to a unique whole (Wu, 2002; NMAP, 2008; Beckmann, 2014). In addition to unpacking CCSSM on
"developing understanding of fractions as numbers," the purpose of this paper is to share fraction tasks implemented in a class of K-8 preservice teachers (PSTs) with the intention of helping third-grade students to focus on the whole when learning fractions.







 The new NCTM website offers many wonderful, FREE resources for its members. Please enjoy the video below to learn more.
The new NCTM website offers many wonderful, FREE resources for its members. Please enjoy the video below to learn more.